Click here and press the right key for the next slide.
(This may not work on mobile or ipad. You can try using chrome or firefox, but even that may fail. Sorry.)
also ...
Press the left key to go backwards (or swipe right)
Press n to toggle whether notes are shown (or add '?notes' to the url before the #)
Press m or double tap to slide thumbnails (menu)
Press ? at any time to show the keyboard shortcuts
Speed-Accuracy Trade-Offs (in Physical Cognition)

Speed-Accuracy Trade-Offs (in Physical Cognition)
s.butterfill@warwick.ac.uk & c.sinigaglia
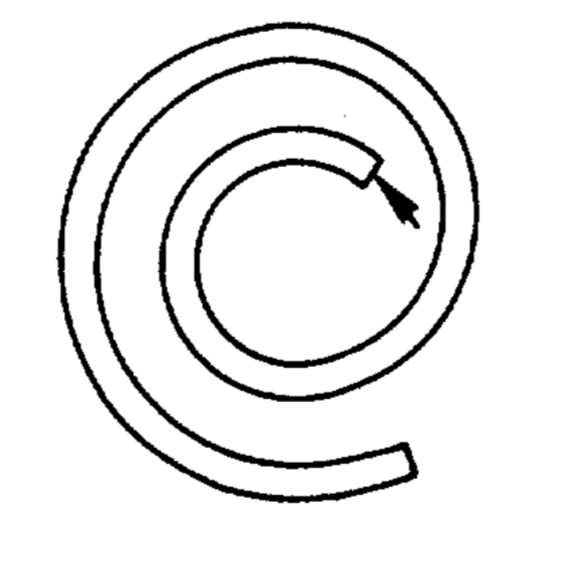
McCloskey, Caramazza, & Green (1980, p. figure 2B)
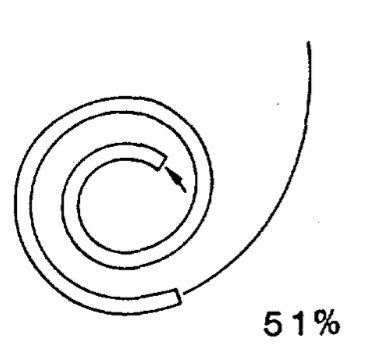
McCloskey et al. (1980, p. figure 2D)
why?
because fast processes make it appear so
(Kozhevnikov & Hegarty, 2001)
background: representational momentum

Hubbard 2005, figure 1a; redrawn from Freyd and Finke 1984, figure 1
Hubbard 2005, figure 1b; drawn from Freyd and Finke 1984, table 1
There are fast process in you
which predict
the future trajectories of physical objects
and they influence perceptual experience.
Next question:
What principles underpin these predictions?
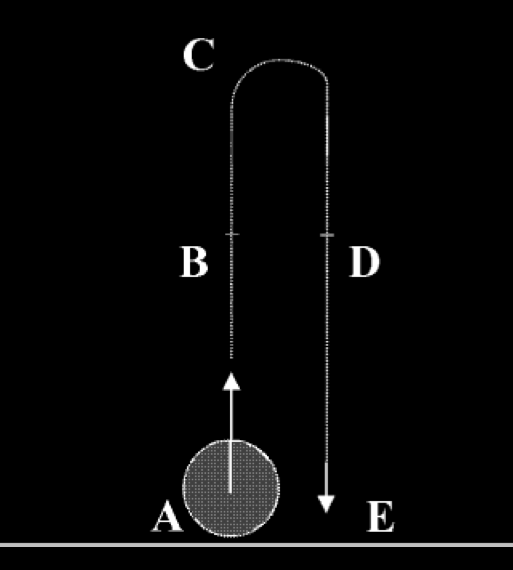
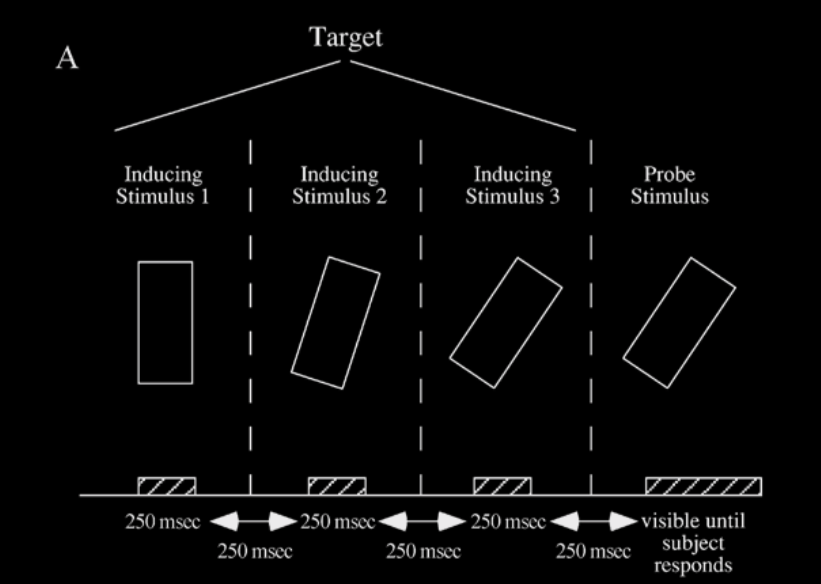
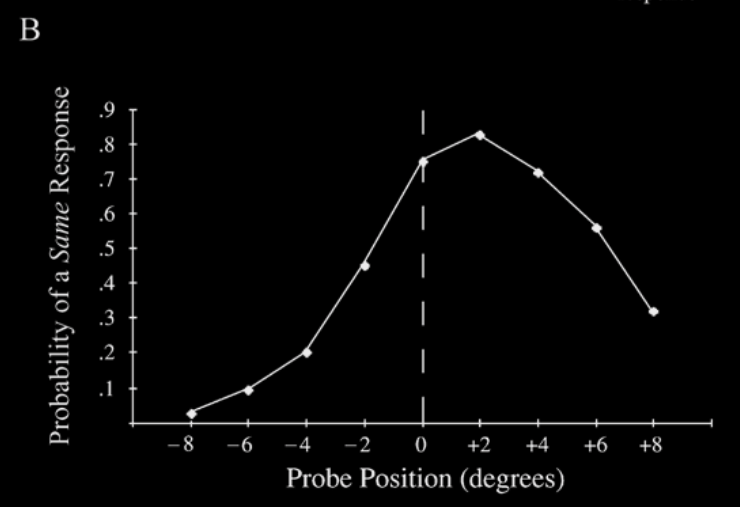
Kozhevnikov & Hegarty (2001, figure 1)
Fix shape and density.
How would increasing the object’s size affect how quickly it decelerates when launched vertically?
Impetus prediction: larger size entails greater deceleration (so slower ascent).
Newtonian prediction: larger size entails lower deceleration (so faster ascent) if considering air resistance; otherwise size makes no difference.
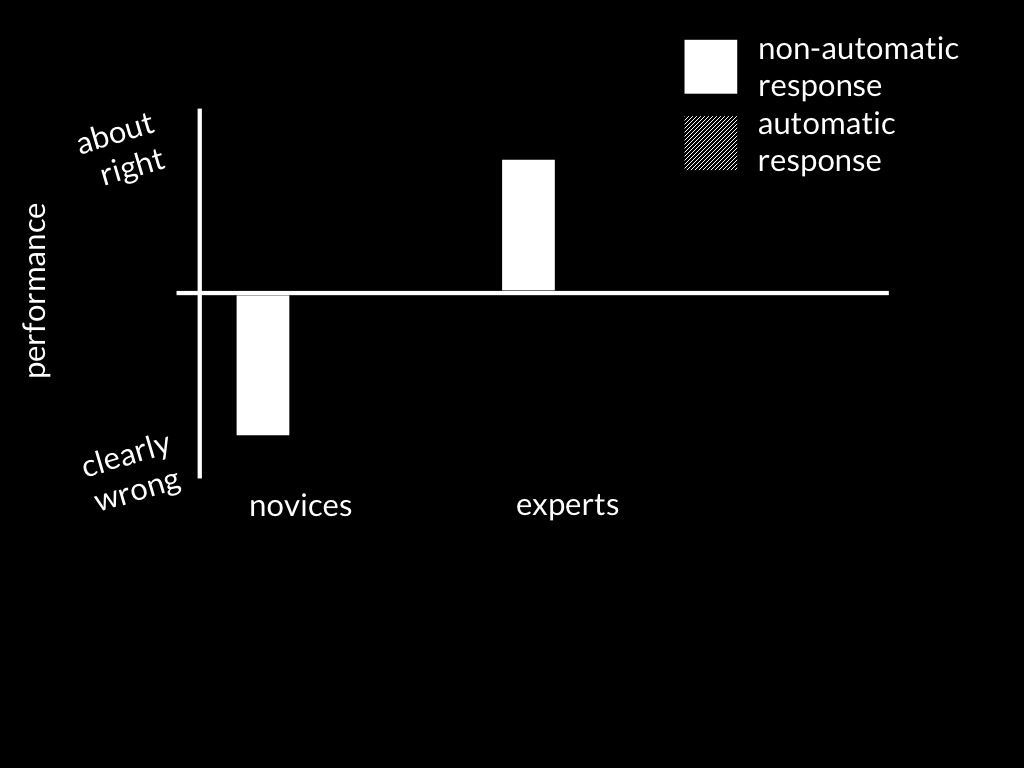
simplified from Kozhevnikov & Hegarty (2001)
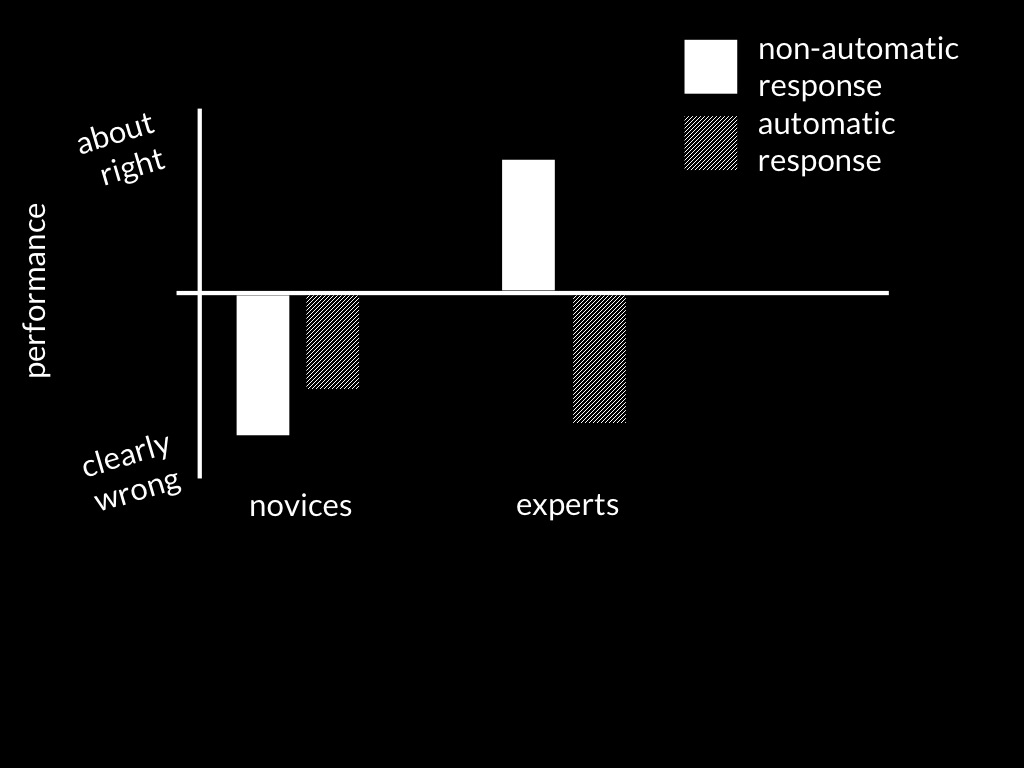
simplified from Kozhevnikov & Hegarty (2001)

McCloskey et al. (1980, p. figure 2D)
why?
because fast processes make it appear so
(Kozhevnikov & Hegarty, 2001)
significance
connecting fast and slow processes
How do fast processes influence slow processes?
ex: explicit verbal judgements
fast process ~ representational momentum
-> phenomenology of experience
-> thinking about experience ~ slow process
-> explicit verbal judgement
phenomenology connects fast and slow indirectly
, leaving room for discretion.
significance
speed vs accuracy trade-off
How can you trade away accuracy to gain speed?
‘To extrapolate objects’ motion on the basis of [e.g. Newtonian] physical principles, one should have assessed and evaluated the presence and magnitude of such imperceptible forces as friction and air resistance ... This would require a time-consuming analysis that is not always possible.
‘In order to have a survival advantage, the process of extrapolation should be fast and effortless, without much conscious deliberation.
‘Impetus theory allows us to extrapolate objects’ motion quickly and without large demands on attentional resources.’
Kozhevnikov and Heggarty (2001, p. 450)
How can you trade away accuracy to gain speed?
By using a simpler model!
| process | model (physics) | model (ethics) |
| fast | impetus | Thomson-esque |
| slow | Newtonian | ??? |
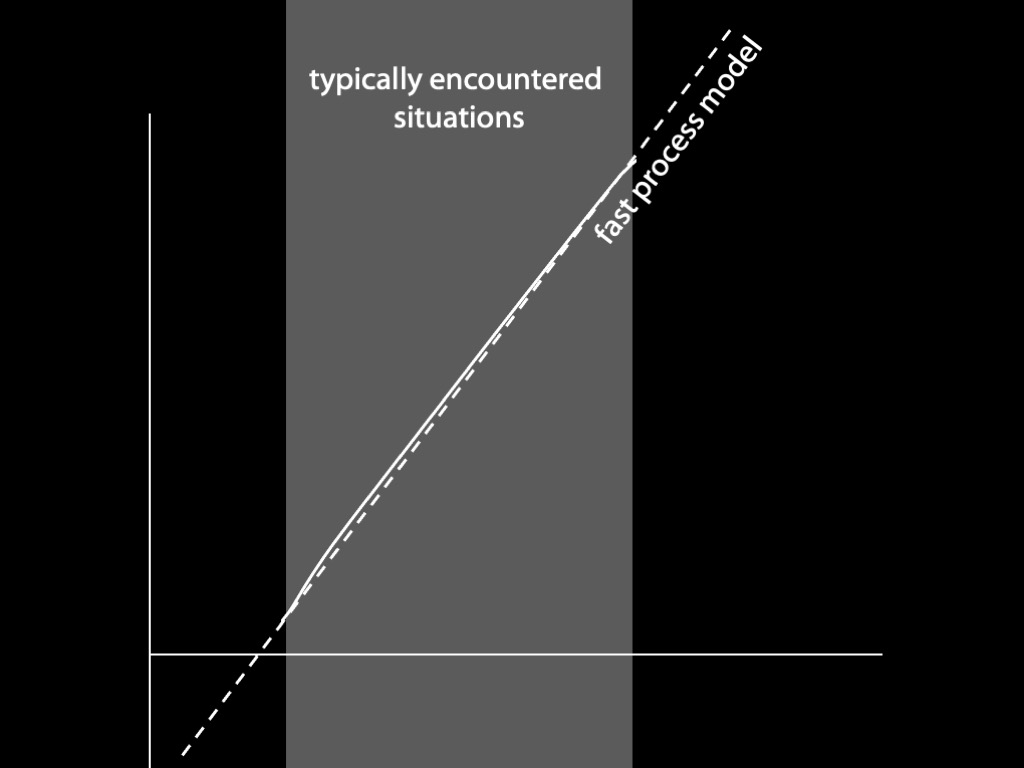
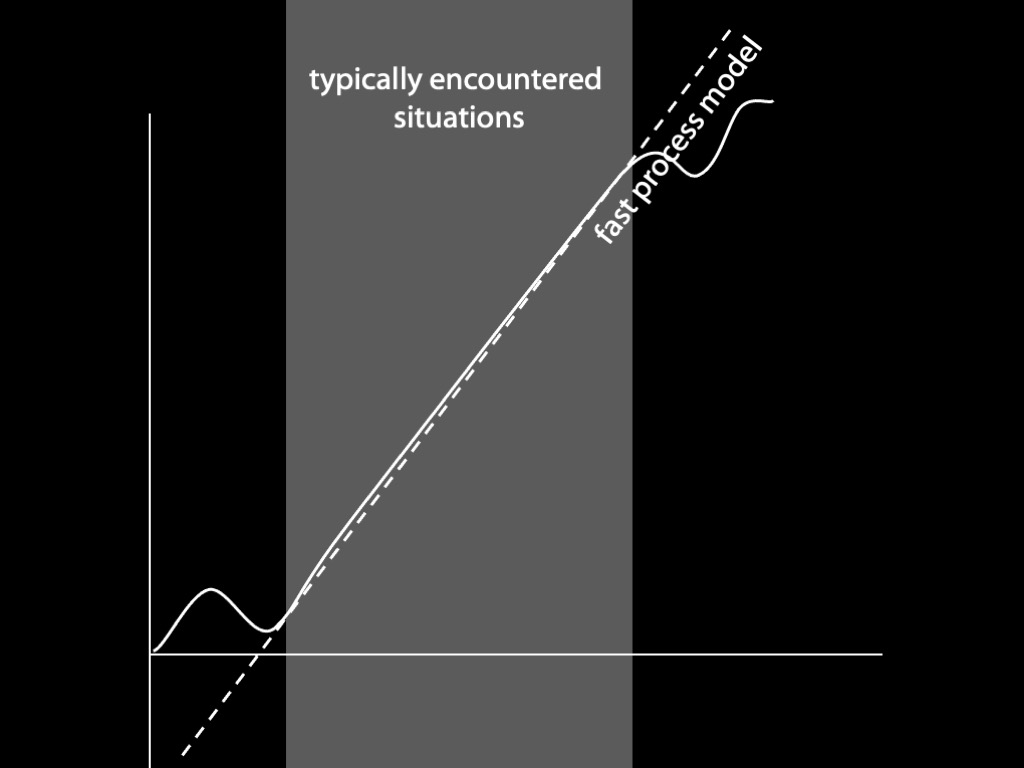
question 1
Why, if at all, are two systems better than one?
question 2
If being able to make
complementary speed-accuracy trade-offs
is one of the reasons why we have two systems,
which properties should we expect
to differentiate the two systems?
implicit / modular
/ ‘system-1’ / ...
innate
informationally encapsulated
domain specific
subject to limited accessibility
speedy
tacit
subpersonal
unconscious
...
‘it seems doubtful that the often long lists of correlated attributes should come as a package’
Adolphs (2010 p. 759)
‘we wonder whether the dichotomous characteristics … are … perfectly correlated
Keren and Schul (2009, p. 537)

conclusion
There is evidence for fast and slow processes in physical cognition.
How do fast process influence slow judgements? Fast process can influence slow judgements via phenomenology.
Fast processes trade accuracy for speed; slow conversely.
Why are two systems better than one? Perhaps because they can make complementary speed-accuracy trade-offs.